All solids, both crystalline and amorphous, have the property of changing their shape under the influence of force applied to them. In other words, they are subject to deformation. If a body returns to its original size and shape after the external force ceases its influence, then it is called elastic, and its deformation is considered elastic. For any body, there is a limit to the applied force, after which the deformation ceases to be elastic, the body does not return to its original shape and original dimensions, but remains in a deformed state or collapses. The theory of elastic deformations of bodies was created at the end of the 17th century by the British scientist R. Hooke and developed in the works of his compatriot Thomas Young. Hooke's and Young's law and coefficient, which determine the degree of elasticity of bodies, were named in their honor, respectively. It is actively used in engineering during calculations of the strength of structures and products.
Basic information
Young's modulus (also called the longitudinal modulus of elasticity and the modulus of elasticity of the first kind) is an important mechanical characteristic of a substance. It is a measure of resistance to longitudinal deformation and determines the degree of rigidity. It is designated as E; measured in n/m 2 or in Pa.
This important coefficient is used when calculating the rigidity of workpieces, assemblies and structures, and in determining their resistance to longitudinal deformations. Substances used for the manufacture of industrial and building structures, as a rule, have very large E values. And therefore, in practice, E values for them are given in gigaPascals (10 12 Pa)
The value of E for rods can be calculated; for more complex structures it is measured during experiments.
Approximate values of E can be obtained from the graph constructed during tensile tests.
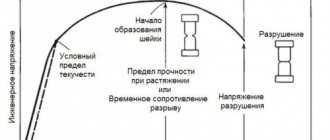
Tensile test schedule
E is the quotient of normal stress σ divided by relative elongation ε.
Hooke's law can also be formulated using Young's modulus.
Tension diagram
To study tensile deformation, a rod made of the material under study is subjected to tension using special devices (for example, a hydraulic press), and the elongation of the sample and the stress arising in it are measured. Based on the experimental results, a graph of the voltage σ
from relative elongation ε
. This graph is called a stretch diagram (Fig. 10).
Rice. 10
Numerous experiments show that at small deformations the stress σ
directly proportional to the relative elongation ε
(section of
the OA
diagram) – Hooke’s law is fulfilled.
The experiment shows that small deformations completely disappear after the load is removed (elastic deformation is observed). At small deformations, Hooke's law is satisfied. The maximum voltage at which Hooke's law still holds is called the limit of proportionality
σп
.
It corresponds to point A
of the diagram.
If you continue to increase the tensile load and exceed the proportional limit, the deformation becomes nonlinear (line ABCDEK
). However, with small nonlinear deformations, after removing the load, the shape and dimensions of the body are practically restored (section AB
graphic arts).
The maximum stress at which noticeable residual deformations do not yet occur is called the elastic limit σуп
.
It corresponds to point B
of the diagram. The elastic limit exceeds the proportionality limit by no more than 0.33%. In most cases they can be considered equal.
If the external load is such that stresses arise in the body that exceed the elastic limit, then the nature of the deformation changes (section BCDEK
). After removing the load, the sample does not return to its previous dimensions, but remains deformed, although with a lower elongation than under load (plastic deformation).
Beyond the elastic limit at a certain stress value corresponding to point C
diagrams, elongation increases practically without increasing the load (section CD
diagram is almost horizontal).
This phenomenon is called material flow
.
With a further increase in load, the voltage increases (from point D
), after which a narrowing (“neck”) appears in the least strong part of the sample. Due to the reduction in cross-sectional area (point E
) for further elongation, less stress is needed, but, in the end, destruction of the sample occurs (point
K
).
The greatest stress that a sample can withstand without failure is called
tensile strength .
Let's denote it σ
pch (it corresponds to point
E
of the diagram). Its meaning strongly depends on the nature of the material and its processing.
To minimize the possibility of destruction of a structure, the engineer must, when making calculations, allow stresses in its elements that will be only part of the ultimate strength of the material. They are called permissible stresses. The number showing how many times the tensile strength is greater than the permissible stress is called the safety factor
.
Denoting the safety margin by n, we get: \(~n = \frac{\sigma_{np}}{\sigma}\) .
The safety factor is selected depending on many reasons: the quality of the material, the nature of the load (static or changing over time), the degree of danger arising from destruction, etc. In practice, the safety factor ranges from 1.7 to 10. By choosing the correct safety factor, the engineer can determine the allowable stress in the structure.
Physical meaning of Young's modulus
During a forced change in the shape of objects, forces are generated inside them that resist such a change and strive to restore the original shape and size of elastic bodies.
If the body does not resist changing shape and remains in a deformed form after the end of the impact, then such a body is called absolutely inelastic, or plastic. A typical example of a plastic body is a block of plasticine.
R. Hooke studied the elongation of rods made of various substances under the influence of weights suspended from the free end. The quantitative expression of the degree of change in shape is considered relative elongation, equal to the ratio of absolute elongation and original length.
As a result of a series of experiments, it was found that absolute elongation is proportional with the elasticity coefficient to the original length of the rod and the deforming force F and inversely proportional to the cross-sectional area of this rod S:
The inverse of α is called Young’s modulus:
ε = (Δl) / l = α * (F/S)
The ratio of tensile force F to S is called elastic stress σ:
Hooke's law, written using Young's modulus, looks like this:
Now we can formulate the physical meaning of Young’s modulus: it corresponds to the stress caused by stretching a rod-shaped sample twice, provided its integrity is maintained.
In reality, the vast majority of samples fail before they have stretched to twice their original length. The value of E is calculated using the indirect method for small deformations.
Stiffness coefficient for elastic deformation of the rod along its axis k = (ES) / l
Young's modulus determines the magnitude of the potential energy of bodies or media subjected to elastic deformation.
Elastic limit
According to the definition, the modulus of elasticity shows how much stress must be applied to a solid body so that its deformation is 100%. However, all solids have an elastic limit, which is equal to 1% deformation. This means that if an appropriate force is applied and the body is deformed by an amount less than 1%, then after the cessation of this force the body exactly restores its original shape and size. If too much force is applied, at which the deformation value exceeds 1%, after the cessation of the external force, the body will no longer restore its original dimensions. In the latter case, they speak of the existence of residual deformation, which is evidence of exceeding the elastic limit of a given material.
Young's modulus values for some materials
The table shows the E values of a number of common substances.
| Material | Young's modulus E, GPa |
| Aluminum | 70 |
| Bronze | 75-125 |
| Tungsten | 350 |
| Graphene | 1000 |
| Brass | 95 |
| Ice | 3 |
| Copper | 110 |
| Lead | 18 |
| Silver | 80 |
| Gray cast iron | 110 |
| Steel | 200/210 |
| Glass | 70 |
The longitudinal elastic modulus of steel is twice the Young's modulus of copper or cast iron. Young's modulus is widely used in formulas for strength calculations of structural elements and products in general.
Design resistances and elastic moduli for building materials
Material
| design resistance of building materials | |
| When calculating building structures, you need to know the design resistance and modulus of elasticity for a particular material. Here is data on the main building materials. Table 1. Elastic moduli for basic building materials | |
| Elastic modulus E, MPa | |
| Cast iron white, gray | (1,15. 1,60) · 10 5 |
| Malleable cast iron | 1,55 · 10 5 |
| Carbon steel | (2,0. 2,1) · 10 5 |
| Alloy steel | (2,1. 2,2) · 10 5 |
| Rolled copper | 1,1 · 10 5 |
| Cold drawn copper | 1,3 · 10 3 |
| Cast copper | 0,84 · 10 5 |
| Rolled phosphor bronze | 1,15 · 10 5 |
| Rolled manganese bronze | 1,1 · 10 5 |
| Cast aluminum bronze | 1,05 · 10 5 |
| Cold drawn brass | (0,91. 0,99) · 10 5 |
| Rolled ship brass | 1,0 · 10 5 |
| Rolled aluminum | 0,69 · 10 5 |
| Aluminum wire drawn | 0,7 · 10 5 |
| Rolled duralumin | 0,71 · 10 5 |
| Rolled zinc | 0,84 · 10 5 |
| Lead | 0,17 · 10 5 |
| Ice | 0,1 · 10 5 |
| Glass | 0,56 · 10 5 |
| Granite | 0,49 · 10 5 |
| Lime | 0,42 · 10 5 |
| Marble | 0,56 · 10 5 |
| Sandstone | 0,18 · 10 5 |
| Granite masonry | (0,09. 0,1) · 10 5 |
| Brick masonry | (0,027. 0,030) · 10 5 |
| Concrete (see table 2) | |
| Wood along the grain | (0,1. 0,12) · 10 5 |
| Wood across the grain | (0,005. 0,01) · 10 5 |
| Rubber | 0,00008 · 10 5 |
| Textolite | (0,06. 0,1) · 10 5 |
| Getinax | (0,1. 0,17) · 10 5 |
| Bakelite | (2. 3) · 10 3 |
| Celluloid | (14,3. 27,5) · 10 2 |
Standard data for calculations of reinforced concrete structures
Table 2. Elastic moduli of concrete (according to SP 52-101-2003)
| Values of the initial modulus of elasticity of concrete in compression and tension Eb, MPa 10 -3, with a concrete class for compressive strength | ||||||||||
| B10 | B15 | B20 | B25 | B30 | B35 | B40 | B45 | B50 | B55 | B60 |
| 19,0 | 24,0 | 27,5 | 30,0 | 32,5 | 34,5 | 36,0 | 37,0 | 38,0 | 39,0 | 39,5 |
Table 2.1 Modulus of elasticity of concrete according to SNiP 2.03.01-84*(1996)
Notes: 1. Above the line the values are indicated in MPa, below the line - in kgf/cm². 2. For lightweight, cellular and porous concrete at intermediate values of concrete density, the initial elastic moduli are taken by linear interpolation. 3. For non-autoclaved cellular concrete, the Eb values are taken as for autoclaved concrete, multiplied by a factor of 0.8. 4. For prestressing concrete, the values of Eb are taken as for heavy concrete, multiplied by coefficient a
= 0.56 + 0.006V.
Table 3. Standard values of concrete resistance (according to SP 52-101-2003)
Table 4. Calculated values of concrete compression resistance (according to SP 52-101-2003)
Table 4.1 Calculated values of concrete compression resistance according to SNiP 2.03.01-84*(1996)
Table 5. Calculated values of concrete tensile strength (according to SP 52-101-2003)
Table 6. Standard resistances for fittings (according to SP 52-101-2003)
Table 6.1 Standard resistances for class A fittings according to SNiP 2.03.01-84* (1996)
Table 6.2 Standard resistances for fittings of classes B and K according to SNiP 2.03.01-84* (1996)
Table 7. Design resistances for reinforcement (according to SP 52-101-2003)
Table 7.1 Design resistances for class A fittings according to SNiP 2.03.01-84* (1996)
Table 7.2 Design resistances for fittings of classes B and K according to SNiP 2.03.01-84* (1996)
Standard data for calculations of metal structures
Table 8. Standard and calculated resistances in tension, compression and bending (according to SNiP II-23-81 (1990)) of sheet, wide-band universal and shaped rolled products in accordance with GOST 27772-88 for steel structures of buildings and structures
Notes: 1. The thickness of the shaped steel should be taken as the thickness of the flange (its minimum thickness is 4 mm). 2. The standard values of the yield strength and tensile strength in accordance with GOST 27772-88 are taken as standard resistance. 3. The values of the calculated resistances are obtained by dividing the standard resistances by the reliability factors for the material, rounded to 5 MPa (50 kgf/cm²).
Table 9. Steel grades replaced by steels according to GOST 27772-88 (according to SNiP II-23-81 (1990))
Notes: 1. Steels S345 and S375 of categories 1, 2, 3, 4 according to GOST 27772-88 replace steels of categories 6, 7 and 9, 12, 13 and 15 according to GOST 19281-73* and GOST 19282-73*, respectively. 2. Steels S345K, S390, S390K, S440, S590, S590K according to GOST 27772-88 replace the corresponding steel grades of categories 1-15 according to GOST 19281-73* and GOST 19282-73*, indicated in this table. 3. Replacement of steels in accordance with GOST 27772-88 with steels supplied in accordance with other state all-Union standards and technical conditions is not provided.
Design resistances for steel used for the production of profiled sheets are not shown here.
Material tensile strength
This is the limit of the stress that occurs, after which the sample begins to fail.
Static tensile strength is measured under prolonged application of deforming force, dynamic - under short-term, impact nature of such force. For most substances the dynamic limit is greater than the static limit.
Read also: Melting point of bronze in degrees
Tensile strength tool
In addition, there are limits to the material's compressive and tensile strength. They are determined experimentally on a test bench, by stretching or compressing samples with powerful hydraulic machines equipped with precise dynamometers and pressure meters. If it is impossible to achieve the required pressure hydraulically, a directed explosion in a sealed capsule is sometimes used.
System stiffness calculation
There are more complex problems in which it is necessary to calculate the total stiffness. In such applications, the springs are connected in series or in parallel.
Series connection of spring system
With a series connection, the overall rigidity of the system decreases. The formula for calculating the elasticity coefficient will be as follows:
1/k = 1/k1 + 1/k2 + … + 1/ki,
where k is the overall stiffness of the system, k1, k2, ..., ki are the individual stiffnesses of each element, i is the total number of all springs involved in the system.
Parallel connection of spring system
In the case when the springs are connected in parallel, the value of the overall elasticity coefficient of the system will increase. The formula for calculation will look like this:
k = k1 + k2 + … + ki.
Measurement of spring stiffness experimentally - in this video.
https://youtube.com/watch?v=YjfWehCZnf8
Allowable tensile stress in some materials
From life experience we know that different materials resist changes in shape in different ways. The strength characteristics of crystalline and other solids are determined by the forces of interatomic interaction. As interatomic distances increase, the forces that attract atoms to each other also increase. These forces reach a maximum at a certain amount of stress, equal to approximately one tenth of Young's modulus.
Tensile test
This value is called theoretical strength; when it is exceeded, the destruction of the material begins. In reality, destruction begins at lower values, since the structure of real samples is heterogeneous. This causes an uneven distribution of stresses, and destruction begins from those areas where the stresses are maximum.
| Materials | σrast | |
| Bor | 5700 | 0,083 |
| Graphite | 2390 | 0,023 |
| Sapphire | 1495 | 0,030 |
| Steel wire | 415 | 0,01 |
| Fiberglass | 350 | 0,034 |
| Structural steel | 60 | 0,003 |
| Nylon | 48 | 0,0025 |
These numbers are taken into account by designers when choosing the material for parts of a future product. Strength calculations are also carried out using them. For example, cables used for lifting and transport operations must have a tenfold safety margin. They are periodically checked by hanging a load ten times greater than the cable's rated load capacity.
The safety margins built into critical structures are also multiple.
Mechanical properties
Only when working in tension or compression does the (Young's) modulus of elasticity help predict the behavior of a particular material . But for bending, shearing, crushing and other loads, you will need to enter additional parameters:
- Stiffness is the product of the profile cross-section and the elastic modulus. By this value one can judge the plasticity of the structural unit as a whole, and not about the material separately. The unit of measurement is kilograms force.
- Longitudinal elongation is the ratio of the absolute elongation of the sample material to its total length. For example, some force was applied to a rod whose length is 200 millimeters. As a result, it became shorter by 5 millimeters. As a result, the relative elongation will be equal to 0.05. This quantity is dimensionless. For more convenient perception, it is sometimes converted into percentages.
- Transverse relative elongation is calculated in the same way as longitudinal relative elongation, but instead of length, the diameter of the rod is taken. It was experimentally found that for a larger amount of material, the transverse elongation is approximately 4 times less than the longitudinal elongation.
- Poisson's ratio. This is the ratio of relative longitudinal to relative transverse strain. Using this value, it is possible to fully describe the changes in shape under the influence of load.
- The shear modulus describes the elastic properties under the influence of tangential properties on the sample. In other words, when the force vector is directed towards the surface of the body at 90 degrees. An example of such loads is the work of nails under crushing, rivets under shear, etc. This parameter is related to the viscosity of the material.
- The bulk modulus of elasticity characterizes the change in the volume of a sample for a versatile uniform application of load. This value is the ratio of volumetric pressure to volumetric compressive strain. As an example, we can consider a material lowered into water, which is subject to liquid pressure over its entire area.
In addition to all of the above, it is worth mentioning that some materials have different mechanical properties depending on the direction of the load . Such materials are called anisotropic. Examples of this are fabrics, some types of stone, laminated plastics, wood, etc.
Isotropic materials have the same mechanical properties and elastic deformation in any direction. Such materials include metals: aluminum, copper, cast iron, steel, etc., as well as rubber, concrete, natural stones, non-laminated plastics.
Safety factor
To quantify the safety factor during design, a safety factor is used. It characterizes the product’s ability to withstand overloads above rated loads. For household products it is small, but for critical components and parts that, if destroyed, can pose a danger to human life and health, it is made multiple times.
Accurate calculation of strength characteristics makes it possible to create a sufficient safety margin and at the same time not to overweight the structure, worsening its performance characteristics. For such calculations, complex mathematical methods and sophisticated software are used. The most important designs are calculated on supercomputers.
Areas of copper use
Due to its mechanical properties, copper has found wide application in various industries, but most often it can be found as an integral part of electrical wires, in heating and air cooling systems, in the production of computer equipment, and heat exchangers.
Industry uses thousands of tons of copper annually
In construction, this metal is used in the manufacture of various structures; the main advantage here is the low volumetric weight of copper. As noted above, non-ferrous metal has found wide application in roofing work, as well as in the manufacture of pipes. The resulting pipes are lightweight and can be transformed, which is especially important when designing water supply and sewerage systems.
The main part of the production of copper products is wire used as a core for electrical or communication cables. Due to the main characteristic of copper - electrical conductivity, it has a high resistance to current, and also has unique magnetic properties - unlike other metals, its particles do not react to a magnet, which sometimes complicates the process of cleaning it. It is worth noting that almost all production of products is based on the processing of secondary raw materials; ore is used extremely rarely.
Relationship with other elastic moduli
Young's modulus is related to the shear modulus, which determines the ability of a sample to resist shear deformation, by the following relationship:
E is also associated with the bulk modulus of elasticity, which determines the sample’s ability to resist simultaneous compression from all sides.
If you find an error, please select a piece of text and press Ctrl+Enter.
One of the main tasks of engineering design is the choice of structural material and optimal profile section. It is necessary to find the size that, with the minimum possible mass, will ensure that the system maintains its shape under load.
For example, what number of steel I-beam should be used as a span beam for a structure? If we take a profile with dimensions smaller than required, we are guaranteed to get the destruction of the structure. If it is more, then this leads to irrational use of metal, and, consequently, heavier construction, more complicated installation, and increased financial costs. Knowledge of such a concept as the modulus of elasticity of steel will answer the above question and will allow you to avoid the occurrence of these problems at a very early stage of production.
Unit of measurement and formulas
The SI unit of Young's modulus is Newton per meter squared (N/m²), i.e. Pascal (Pa).
Formulas
There are several formulas from which you can calculate Young's modulus. For example, Hooke's law.
Hooke's law
You can calculate Young's modulus using these formulas (we will do this using an example). Because of this law, there are several interesting equalities that can be useful for calculations.
Hooke's law (this one describes phenomena in the body, in differential form):
- σ - mechanical stress
- E - Young's modulus (elastic modulus)
- ε—relative elongation
Hooke's Law (this one describes phenomena in the body)
- Fupr - elastic force
- k × Δl—body elongation
- Fupr - elastic force
- E - Young's modulus (elastic modulus)
- S - cross-sectional area
- l - initial body length
- Δl - body elongation
- Fpr/S - mechanical stress, denoted as σ
- Δl/l - relative elongation, denoted as ε
It should be noted that this law applies to the point where the material is irreversibly deformed and no longer returns to its original shape. At what point this happens depends on the material. If the material is very stiff (meaning a high elastic modulus reading), then this point may coincide with rupture/deformation.
Other formulas for calculating Young's modulus (elastic modulus)
- E - Young's modulus (elastic modulus)
- k - body stiffness
- l is the original length of the rod
- S - cross-sectional area
Or you can express k (body stiffness):
- k - body stiffness
- E - Young's modulus (elastic modulus)
- S - cross-sectional area
- l is the initial length of the rod/body
An example of solving the problem (via Hooke's law):
A wire 2.5 meters long and with a cross-sectional area of 2.5 millimeters² is elongated by 1 millimeter under a force of 50 newtons. Determine Young's modulus.
- l = 2.5 m
- F=50H
- E = ?
We will search through Hooke's law (σ = E × ε).
Remember from Hooke's law:
σ = F / S (remember that Fpr/S is mechanical stress, denoted as σ)
ε = Δl/l (and this is the relative elongation, denoted as ε)
Substitute into the formula (σ = E × ε):
For example, in our table, cadmium has such a Young’s modulus.
General concept
The modulus of elasticity (also known as Young's modulus) is one of the indicators of the mechanical properties of a material, which characterizes its resistance to tensile deformation. In other words, its value shows the ductility of the material. The greater the elastic modulus, the less any rod will stretch, all other things being equal (load magnitude, cross-sectional area, etc.).
In the theory of elasticity, Young's modulus is denoted by the letter E. It is an integral part of Hooke's law (the law on the deformation of elastic bodies). Connects the stress arising in the material and its deformation.
According to the international standard system of units, it is measured in MPa. But in practice, engineers prefer to use the dimension kgf/cm2.
The elastic modulus is determined experimentally in scientific laboratories. The essence of this method is to tear dumbbell-shaped samples of material using special equipment. Having found out the stress and elongation at which the sample failed, divide these variables by each other, thereby obtaining Young's modulus.
Let us immediately note that this method is used to determine the elastic moduli of plastic materials: steel, copper, etc. Brittle materials - cast iron, concrete - are compressed until cracks appear.
Additional characteristics of mechanical properties
The modulus of elasticity makes it possible to predict the behavior of a material only when working in compression or tension. In the presence of such types of loads as crushing, shear, bending, etc., additional parameters will need to be introduced:
- Stiffness is the product of the elastic modulus and the cross-sectional area of the profile. By the value of rigidity, one can judge the plasticity not of the material, but of the structure as a whole. Measured in kilograms of force.
- Relative longitudinal elongation shows the ratio of the absolute elongation of the sample to the total length of the sample. For example, a certain force was applied to a rod 100 mm long. As a result, it decreased in size by 5 mm. Dividing its elongation (5 mm) by the original length (100 mm) we obtain a relative elongation of 0.05. A variable is a dimensionless quantity. In some cases, for ease of perception, it is converted to percentages.
- Relative transverse elongation is calculated similarly to the point above, but instead of length, the diameter of the rod is considered here. Experiments show that for most materials, transverse elongation is 3-4 times less than longitudinal elongation.
- The Punch ratio is the ratio of the relative longitudinal strain to the relative transverse strain. This parameter allows you to fully describe the change in shape under the influence of load.
- The shear modulus characterizes the elastic properties when the sample is exposed to tangential stresses, i.e., in the case when the force vector is directed at 90 degrees to the surface of the body. Examples of such loads are the work of rivets in shear, nails in crushing, etc. By and large, the shear modulus is associated with such a concept as the viscosity of the material.
- The bulk modulus of elasticity is characterized by a change in the volume of the material for uniform, versatile application of load. It is the ratio of volumetric pressure to volumetric compressive strain. An example of such work is a sample lowered into water, which is subject to liquid pressure over its entire area.
Read also: What happens to metal when cooled
In addition to the above, it should be mentioned that some types of materials have different mechanical properties depending on the direction of loading. Such materials are characterized as anisotropic. Vivid examples are wood, laminated plastics, some types of stone, fabrics, etc.
Isotropic materials have the same mechanical properties and elastic deformation in any direction. These include metals (steel, cast iron, copper, aluminum, etc.), non-laminated plastics, natural stones, concrete, rubber.
What is copper
Cuprum
One of the most common non-ferrous metals used in industry is copper, its Latin name is Cuprum, after the island of Cyprus, where it was mined by the Greeks many thousands of years ago. This is one of the seven metals that were known in ancient times; jewelry, dishes, money, and tools were made from it.
Historians even called the period (from the 4th to the 3rd millennium BC) the Copper Age. D.I. Mendeleev put this metal in 29th place in his table, after hydrogen, since copper does not displace it from an acidic environment. Copper is a non-ferrous metal that has unique physical, mechanical, and chemical properties.
The density of copper in kg m³ is one of the most important characteristics; it is used to determine the weight of the future product.
How is density determined?
The density of any substance is an indicator of the ratio of mass to total volume. The most common system for measuring density is kilogram per cubic meter. For copper this figure is 8.93 kg/m³. Since there are different grades of metal, which differ depending on the impurities of other substances, the overall density may vary. In this case, it is more appropriate to use another characteristic - specific gravity. In measuring systems, this indicator is expressed in different quantities:
Formula for determining the density of a substance
- SGS system - dyn/cm³;
- SI system - n/m³;
- MKSS system - kg/m³
In this case, the following formula can be used to convert values:
1 n/m³ = 1 dyne/cm³ = 0.102 kg/m³.
Specific gravity is an important indicator in the production of various materials containing copper, especially when it comes to its alloys. This is the ratio of the mass of copper to the total volume of the alloy.
You can consider how this indicator is used in practice using the example of calculating the weight of 25 copper sheets, 2000*1000 mm in size, 5 mm thick. First, let's determine the volume of the sheet - 5 mm * 2000 mm * 1000 mm = 10000000 mm3 or 10,000 cm³.
Specific gravity of copper 8.94 g/cm³
We calculate the weight of copper in one sheet - 10,000 * 8.94 = 89,400 g or 89.40 kg.
The mass of rolled copper in the total amount of material is 89.40 * 25 = 2,235 kg.
This calculation scheme is also used when processing scrap metal.
Basic properties
Smelting copper from ore
Copper, as a metal, is obtained by smelting ore; in nature it is difficult to find pure nuggets; mainly enrichment and extraction is carried out from:
- chalcocite ore, in which the copper content is about 80%, this type is often called copper luster;
- Bronite ore, here the metal content is up to 65%
- covellite ore - up to 64%.
In terms of its physical properties, copper is a red-colored metal; a pink tint may be present in the section; it is classified as a heavy metal because it has a high density.
A distinctive characteristic is electrical conductivity. Due to this, the metal is widely used in the manufacture of cables and electrical wires. In this indicator, copper is second only to silver; in addition, there are a number of other physical characteristics:
- hardness - on the Brindel scale equals 35 kgf/mm²;
- elasticity - 132000 MN/m²;
- linear thermal expansion - 0.00000017 units;
- relative elongation - 60%;
- melting point - 1083 ºС;
- boiling point - 2600 ºС;
- thermal conductivity coefficient - 335 kcal/m*h*deg.
Elastic modulus value
It should be noted that Young's modulus is not a constant value. Even for the same material, it can fluctuate depending on the points at which the force is applied.
Some elastic-plastic materials have a more or less constant modulus of elasticity when working in both compression and tension: copper, aluminum, steel. In other cases, the elasticity may vary based on the shape of the profile.
Here are examples of Young's modulus values (in millions kgscm2) of some materials:
- White cast iron – 1.15.
- Gray cast iron -1.16.
- Brass – 1.01.
- Bronze – 1.00.
- Brick masonry - 0.03.
- Granite stonework - 0.09.
- Concrete – 0.02.
- Wood along the grain – 0.1.
- Wood across the grain – 0.005.
- Aluminum – 0.7.
Let's consider the difference in readings between elastic moduli for steels depending on the grade:
- High quality structural steel (20, 45) – 2.01.
- Standard quality steel (St. 3, St. 6) – 2.00.
- Low alloy steels (30ХГСА, 40Х) – 2.05.
- Stainless steel (12Х18Н10Т) – 2.1.
- Die steel (9ХМФ) – 2.03.
- Spring steel (60С2) – 2.03.
- Bearing steel (ШХ15) – 2.1.
Also, the value of the elastic modulus for steels varies depending on the type of rolled product:
- High strength wire – 2.1.
- Braided rope – 1.9.
- Cable with a metal core - 1.95.
As we can see, the deviations between steels in the values of elastic deformation moduli are small. Therefore, in most engineering calculations, errors can be neglected and the value E = 2.0 taken.
The development of metallurgy and other related areas for the production of metal objects is due to the creation of weapons. At first they learned to smelt non-ferrous metals, but the strength of the products was relatively low. Only with the advent of iron and its alloys did the study of their properties begin.
The first swords were made quite heavy to give them hardness and strength. Warriors had to take them in both hands to control them. Over time, new alloys appeared and production technologies were developed. Light sabers and swords came to replace heavy weapons. At the same time, tools were created. With increasing strength characteristics, tools and production methods were improved.
Young's modulus of elasticity and shear, Poisson's ratio values (Table)
Elastic properties of bodies
Below are reference tables for commonly used constants; if two of them are known, then this is quite sufficient to determine the elastic properties of a homogeneous isotropic solid.
Young's modulus or modulus of longitudinal elasticity in dyn/cm2.
Shear modulus or torsional modulus G in dyn/cm2.
Compressive modulus or bulk modulus K in dynes/cm2.
Compressibility volume k=1/K/.
Poisson's ratio µ is equal to the ratio of transverse relative compression to longitudinal relative tension.
For a homogeneous isotropic solid material, the following relationships between these constants hold:
G = E / 2(1 + μ) - (α)
μ = (E / 2G) - 1 - (b)
K = E / 3(1 - 2μ) - (c)
Poisson's ratio has a positive sign and its value is usually between 0.25 and 0.5, but in some cases it can go beyond these limits. The degree of agreement between the observed values of µ and those calculated using formula (b) is an indicator of the isotropy of the material.
Tables of Young's Modulus of Elasticity, Shear Modulus and Poisson's Ratio
Values calculated from relations (a), (b), (c) are given in italics.
| Material at 18°C | Young's modulus E, 1011 dynes/cm2. | Shear modulus G, 1011 dynes/cm2. | Poisson's ratio µ | Modulus of bulk elasticity K, 1011 dynes/cm2. |
| Aluminum | 7,05 | 2,62 | 0,345 | 7,58 |
| Bismuth | 3,19 | 1,20 | 0,330 | 3,13 |
| Iron | 21,2 | 8,2 | 0,29 | 16,9 |
| Gold | 7,8 | 2,7 | 0,44 | 21,7 |
| Cadmium | 4,99 | 1,92 | 0,300 | 4,16 |
| Copper | 12,98 | 4,833 | 0,343 | 13,76 |
| Nickel | 20,4 | 7,9 | 0,280 | 16,1 |
| Platinum | 16,8 | 6,1 | 0,377 | 22,8 |
| Lead | 1,62 | 0,562 | 0,441 | 4,6 |
| Silver | 8,27 | 3,03 | 0,367 | 10,4 |
| Titanium | 11,6 | 4,38 | 0,32 | 10,7 |
| Zinc | 9,0 | 3,6 | 0,25 | 6,0 |
| Steel (1% C) 1) | 21,0 | 8,10 | 0,293 | 16,88 |
| (soft) | 21,0 | 8,12 | 0,291 | 16,78 |
| Constantan 2) | 16,3 | 6,11 | 0,327 | 15,7 |
| Manganin | 12,4 | 4,65 | 0,334 | 12,4 |
| 1) For steel containing about 1% C, elastic constants are known to change during heat treatment. 2) 60% Cu, 40% Ni. |
The experimental results given below are for common laboratory materials, mainly wires.
| Substance | Young's modulus E, 1011 dynes/cm2. | Shear modulus G, 1011 dynes/cm2. | Poisson's ratio µ | Modulus of bulk elasticity K, 1011 dynes/cm2. |
| Bronze (66% Cu) | -9,7-10,2 | 3,3-3,7 | 0,34-0,40 | 11,2 |
| Copper | 10,5-13,0 | 3,5-4,9 | 0,34 | 13,8 |
| Nickel silver1) | 11,6 | 4,3-4,7 | 0,37 | — |
| Glass | 5,1-7,1 | 3,1 | 0,17-0,32 | 3,75 |
| Glass yen crowns | 6,5-7,8 | 2,6-3,2 | 0,20-0,27 | 4,0-5,9 |
| Jena flint glass | 5,0-6,0 | 2,0-2,5 | 0,22-0,26 | 3,6-3,8 |
| Welding iron | 19-20 | 7,7-8,3 | 0,29 | 16,9 |
| Cast iron | 10-13 | 3,5-5,3 | 0,23-0,31 | 9,6 |
| Magnesium | 4,25 | 1,63 | 0,30 | — |
| Phosphor bronze2) | 12,0 | 4,36 | 0,38 | — |
| Platinoid3) | 13,6 | 3,6 | 0,37 | — |
| Quartz threads (floating) | 7,3 | 3,1 | 0,17 | 3,7 |
| Soft vulcanized rubber | 0,00015-0,0005 | 0,00005-0,00015 | 0,46-0,49 | — |
| Steel | 20-21 | 7,9-8,9 | 0,25-0,33 | 16,8 |
| Zinc | 8,7 | 3,8 | 0,21 | — |
| 1) 60% Cu, 15% Ni, 25% Zn 2) 92.5% Cu, 7% Sn, 0.5% P 3) Nickel silver with a small amount of tungsten. |
| Substance | Young's modulus E, 1011 dynes/cm2. | Substance | Young's modulus E, 1011 dynes/cm2. |
| Zinc (pure) | 9,0 | Oak | 1,3 |
| Iridium | 52,0 | Pine | 0,9 |
| Rhodium | 29,0 | Red tree | 0,88 |
| Tantalum | 18,6 | Zirconium | 7,4 |
| Invar | 17,6 | Titanium | 10,5-11,0 |
| Alloy 90% Pt, 10% Ir | 21,0 | Calcium | 2,0-2,5 |
| Duralumin | 7,1 | Lead | 0,7-1,6 |
| Silk threads1 | 0,65 | Teak | 1,66 |
| Web2 | 0,3 | Silver | 7,1-8,3 |
| Catgut | 0,32 | Plastics: | |
| Ice (-20C) | 0,28 | Thermoplastic | 0,14-0,28 |
| Quartz | 7,3 | Thermoset | 0,35-1,1 |
| Marble | 3,0-4,0 | Tungsten | 41,1 |
| 1) Reduces rapidly with increasing load 2) Detects noticeable elastic fatigue |
| Temperature coefficient (at 150C) Et=E11 (1-ɑ (t-15)), Gt=G11 (1-ɑ (t-15)) | Compressibility k, bar-1 (at 7-110C) | |||
| ɑ, for E | ɑ, for G | |||
| Aluminum | 4,8*10-4 | 5,2*10-4 | Aluminum | 1,36*10-6 |
| Brass | 3,7*10-4 | 4,6*10-4 | Copper | 0,73*10-6 |
| Gold | 4,8*10-4 | 3,3*10-4 | Gold | 0,61*10-6 |
| Iron | 2,3*10-4 | 2,8*10-4 | Lead | 2,1*10-6 |
| Steel | 2,4*10-4 | 2,6*10-4 | Magnesium | 2,8*10-6 |
| Platinum | 0,98*10-4 | 1,0*10-4 | Platinum | 0,36*10-6 |
| Silver | 7,5*10-4 | 4,5*10-4 | Flint glass | 3,0*10-6 |
| Tin | — | 5,9*10-4 | German glass | 2,57*10-6 |
| Copper | 3,0*10-4 | 3,1*10-4 | Steel | 0,59*10-6 |
| Nickel silver | — | 6,5*10-4 | ||
| Phosphor bronze | — | 3,0*10-4 | ||
| Quartz threads | -1,5*10-4 | -1,1*10-4 |
Types of loads
When using metals, different static and dynamic loads are applied. In the theory of strength, it is customary to define the following types of loads.
- Compression - an acting force compresses an object, causing a decrease in length along the direction of application of the load. This deformation is felt by frames, supporting surfaces, racks and a number of other structures that can withstand a certain weight. Bridges and crossings, car and tractor frames, foundations and reinforcement - all these structural elements are under constant compression.
Read also: How to make wood engraving
- Tension - the load tends to lengthen the body in a certain direction. Lifting and transport machines and mechanisms experience similar loads when lifting and carrying loads.
- Shear and shear - such loading is observed in the case of forces directed along the same axis towards each other. Connecting elements (bolts, screws, rivets and other hardware) experience this type of load. The design of housings, metal frames, gearboxes and other components of mechanisms and machines necessarily contains connecting parts. The performance of devices depends on their strength.
- Torsion - if a pair of forces acting on an object are located at a certain distance from each other, then a torque occurs. These forces tend to produce torsional deformation. Similar loads are observed in gearboxes; the shafts experience just such a load. It is most often inconsistent in meaning. Over time, the magnitude of the acting forces changes.
- Bending – a load that changes the curvature of objects is considered bending. Bridges, crossbars, consoles, lifting and transport mechanisms and other parts experience similar loading.
Basic properties
Smelting copper from ore
Copper, as a metal, is obtained by smelting ore; in nature it is difficult to find pure nuggets; mainly enrichment and extraction is carried out from:
- chalcocite ore, in which the copper content is about 80%, this type is often called copper luster;
- Bronite ore, here the metal content is up to 65%
- covellite ore - up to 64%.
In terms of its physical properties, copper is a red-colored metal; a pink tint may be present in the section; it is classified as a heavy metal because it has a high density.
A distinctive characteristic is electrical conductivity. Due to this, the metal is widely used in the manufacture of cables and electrical wires. In this indicator, copper is second only to silver; in addition, there are a number of other physical characteristics:
- hardness - on the Brindel scale equals 35 kgf/mm²;
- elasticity - 132000 MN/m²;
- linear thermal expansion - 0.00000017 units;
- relative elongation - 60%;
- melting point - 1083 ºС;
- boiling point - 2600 ºС;
- thermal conductivity coefficient - 335 kcal/m*h*deg.
The main properties of copper include the elastic modulus, which is calculated by various methods:
The shear modulus is useful to know in the production of materials for the construction industry - it is a value that characterizes the degree of resistance to shear and deformation under the influence of various loads. The modulus calculated using Young's method shows how the metal will behave under uniaxial tension. The shear modulus characterizes the response of a metal to shear load. Poisson's ratio shows how a material behaves under uniform compression.
Development of mines for the extraction of copper and other metals
The chemical properties of copper describe the combination with other substances into alloys and possible reactions to an acidic environment. The most significant characteristic is oxidation. This process actively manifests itself during heating; already at a temperature of 375 ºC, copper oxide begins to form, or scale as it is called, which can affect the conductive functions of the metal and reduce them.
When copper reacts with a solution of an iron salt, it turns into a liquid state. This method is used to remove copper coating on various products.
Long stay in water causes cuprite
When copper is exposed to a humid environment for a long time, cuprite, a greenish coating, forms on its surface. This property of copper is taken into account when using metal to cover roofs. It is noteworthy that cuprite performs a protective function; the metal underneath does not deteriorate at all, even for a hundred years. The only opponents of copper roofs are environmentalists. They explain their position by the fact that when copper cuprite is washed off by rainwater into the soil or water bodies, it pollutes it with its toxins, which especially has a detrimental effect on microorganisms living in rivers and lakes. But to solve this problem, builders use drainpipes made of a special metal, which absorbs copper particles and accumulates them, while the water flows free of toxins.
Copper sulfate is another result of chemical action on metal. This substance is actively used by agronomists to fertilize the soil and stimulate the growth of various crops. However, uncontrolled use of vitriol can also have a detrimental effect on the environment. Toxins penetrate deep into the layers of the earth and accumulate in groundwater.
The concept of elastic modulus
In the middle of the 17th century, materials research began simultaneously in several countries. A variety of methods have been proposed for determining strength characteristics. The English researcher Robert Hooke (1660) formulated the main provisions of the law on the elongation of elastic bodies as a result of the application of a load (Hooke's law). The following concepts were also introduced:
- Stress σ, which in mechanics is measured in the form of a load applied to a certain area (kgf/cm², N/m², Pa).
- Elastic modulus E, which determines the ability of a solid body to deform under loading (applying force in a given direction). Units of measurement are also defined in kgf/cm² (N/m², Pa).
The formula according to Hooke's law is written as ε = σz/E, where:
- ε – relative elongation;
- σz – normal stress.
Demonstration of Hooke's law for elastic bodies:
From the above dependence, the value of E for a certain material is derived experimentally, E = σz/ε.
In the theory of strength, the concept of Young's modulus of elasticity is adopted. This English researcher gave a more specific description of the methods of changing strength indicators under normal loads.
The elastic modulus values for some materials are given in Table 1.
Table 1: Modulus of elasticity for metals and alloys
| Name of material | Elastic modulus value, 10¹² Pa |
| Aluminum | 65…72 |
| Duralumin | 69…76 |
| Iron, carbon content less than 0.08% | 165…186 |
| Brass | 88…99 |
| Copper (Cu, 99%) | 107…110 |
| Nickel | 200…210 |
| Tin | 32…38 |
| Lead | 14…19 |
| Silver | 78…84 |
| Gray cast iron | 110…130 |
| Steel | 190…210 |
| Glass | 65…72 |
| Titanium | 112…120 |
| Chromium | 300…310 |
What it is?
Elastic modulus (Young's modulus) is an indicator that determines the mechanical response of a material. Using this parameter, it is possible to characterize the behavior of the sample under tension. In simpler terms, the modulus of elasticity means the plastic properties of steel, and the higher the indicator, the lower the elongation. In theory, Young's modulus is denoted by the letter "E". This is one of the components of Hooke's law, which considers possible deformations of elastic bodies. Using this value, it is possible to connect the stresses arising in the material with the deformation that it experiences. The unit of elastic modulus is pascals (Pa) or megapascals (MPa). However, engineers often give preference to kgf/cm2 when carrying out calculations. The indicator is determined through research in laboratories, recording samples on special equipment. The technique is based on breaking samples in the shape of dumbbells on automated installations.
During the experiment, the automation monitors indicators of changes in the length and tension of the workpiece, at which it collapses, and then divides the results. The resulting number will be Young's modulus or elastic modulus. It is noteworthy that a similar method for determining the indicator is used to determine E:
- become;
- copper;
- other elastic samples.
In brittle materials, the parameter is determined by compression until cracks appear. It is worth taking a closer look at the analysis of Young's modulus from the point of view of physics. During the process of forced loading, which leads to a change in the shape of the material, response forces arise within it. Forces begin to resist external stresses and strive to restore the shape of the body. If a sample does not react at all to the load (more precisely, it completely changes its shape and does not restore it when the stress is removed), it is considered to be plastic. As an example, it is worth mentioning plasticine, which clearly reflects theory in practice. The study of the elasticity of materials was carried out by the scientist R. Hooke, who was interested in how rods of different materials would change and lengthen under the influence of weights. Thanks to a previously conducted series of experiments, it was possible to prove that the values of absolute elongation and initial length are directly proportional. At the same time, the absolute elongation is inversely proportional to the cross-sectional area of the rod under study.
Hooke derived a whole law and also introduced the parameter E to characterize the properties of an elastic material. Thus, the physical meaning of the module is that the parameter corresponds to the stress caused in the rod when stretched to a length that is twice as high, provided there is no visible damage to the sample.
Using module E, it is possible to predict how the material will behave under certain loads. However, it does not provide an understanding of what will happen to it under other loading methods. Therefore, to carry out effective calculations, it is necessary to introduce additional parameters.
- Rigidity. The indicator demonstrates the degree of plasticity of the joint of the sample under study. The unit of measurement of the parameter is kgf.
- Relative elongation in the longitudinal direction. Two indicators are used in the calculation: the absolute elongation value and the total length of the sample. The indicator does not have a unit of measurement, but for simplified understanding it is multiplied by 100%.
- Elongation in the transverse direction. It is calculated in the same way as the previous parameter, only instead of the length, the diameter of the sample rod is used. As tests have shown, transverse elongation is usually less than longitudinal elongation.
- Poisson's ratio. It is the ratio of the last two indicators. The parameter makes it possible to describe how the material will change its shape based on the magnitude of the load and the location of its application.
- Shear modulus. With its help, it is possible to describe the behavior of a material with elastic properties when exposed to tangential forces. In other words, it helps to evaluate the performance of a structure when exposed to wind at an angle of 90 degrees.
Elastic modulus for different steel grades
Metallurgists have developed several hundred grades of steel. They have different strength values. Table 2 shows the characteristics for the most common steels.
Table 2: Elasticity of steels
| Name of steel | Elastic modulus value, 10¹² Pa |
| Low carbon steel | 165…180 |
| Steel 3 | 179…189 |
| Steel 30 | 194…205 |
| Steel 45 | 211…223 |
| Steel 40Х | 240…260 |
| 65G | 235…275 |
| X12MF | 310…320 |
| 9ХС, ХВГ | 275…302 |
| 4Х5МФС | 305…315 |
| 3Х3М3Ф | 285…310 |
| R6M5 | 305…320 |
| P9 | 320…330 |
| P18 | 325…340 |
| R12MF5 | 297…310 |
| U7, U8 | 302…315 |
| U9, U10 | 320…330 |
| U11 | 325…340 |
| U12, U13 | 310…315 |
Video: Hooke's law, modulus of elasticity.
Strength modules
In addition to normal loading, there are other force effects on materials.
The shear modulus G determines the stiffness. This characteristic shows the maximum load value for changing the shape of an object.
The bulk modulus of elasticity K determines the elastic properties of a material to change volume. With any deformation, the shape of the object changes.
For different steels, the values of the indicated modules are given in Table 3.
Table 3: Strength moduli for steels
| Name of steel | Young's modulus of elasticity, 10¹² Pa | Shear modulus G, 10¹² Pa | Modulus of bulk elasticity, 10¹² Pa | Poisson's ratio, 10¹²·Pa |
| Low carbon steel | 165…180 | 87…91 | 45…49 | 154…168 |
| Steel 3 | 179…189 | 93…102 | 49…52 | 164…172 |
| Steel 30 | 194…205 | 105…108 | 72…77 | 182…184 |
| Steel 45 | 211…223 | 115…130 | 76…81 | 192…197 |
| Steel 40Х | 240…260 | 118…125 | 84…87 | 210…218 |
| 65G | 235…275 | 112…124 | 81…85 | 208…214 |
| X12MF | 310…320 | 143…150 | 94…98 | 285…290 |
| 9ХС, ХВГ | 275…302 | 135…145 | 87…92 | 264…270 |
| 4Х5МФС | 305…315 | 147…160 | 96…100 | 291…295 |
| 3Х3М3Ф | 285…310 | 135…150 | 92…97 | 268…273 |
| R6M5 | 305…320 | 147…151 | 98…102 | 294…300 |
| P9 | 320…330 | 155…162 | 104…110 | 301…312 |
| P18 | 325…340 | 140…149 | 105…108 | 308…318 |
| R12MF5 | 297…310 | 147…152 | 98…102 | 276…280 |
| U7, U8 | 302…315 | 154…160 | 100…106 | 286…294 |
| U9, U10 | 320…330 | 160…165 | 104…112 | 305…311 |
| U11 | 325…340 | 162…170 | 98…104 | 306…314 |
| U12, U13 | 310…315 | 155…160 | 99…106 | 298…304 |
For other materials, strength characteristics are indicated in specialized literature. However, in some cases individual studies are carried out. Such research is especially relevant for building materials. At enterprises where reinforced concrete products are produced, tests are regularly carried out to determine limit values.
Modulus of deformation of steel and its elasticity
The main task of engineering design is the selection of the optimal profile section and structural material. It is necessary to find exactly the size that will ensure that the shape of the system is maintained with the minimum possible mass under the influence of load. For example, what kind of steel should be used as a span beam for a structure? The material may be used irrationally, installation will become more complicated and the structure will become heavier, and financial costs will increase. This question will be answered by such a concept as the elastic modulus of steel. It will also allow you to avoid these problems at a very early stage.